Introduction
In his mathematical theory of
communication, Shannon detached himself from the communication of meaning by
stating on the first page that the “semantic aspects of communication are
irrelevant to the engineering problem” (Shannon & Weaver 1949, at p. 3). However,
his co-author Weaver noted the following:
The
concept of information developed in this theory at first seems disappointing
and bizarre--disappointing because it has nothing to do with the meaning, and
bizarre because it deals not with a single message but rather with the
statistical character of a whole ensemble of messages, bizarre also because in
these statistical terms the two words information and uncertainty
find themselves to be partners.
I think, however, that these should be only temporary
reactions; and that one should say, at the end, that this analysis has so
penetratingly cleared the air that one is now, perhaps for the first time,
ready for a real theory of meaning. (Ibid., at pp. 116f.)
Recent advances in the computation of anticipatory systems enable
us to model both the generation of meaning by anticipatory agents and meaning-processing
at the supra-individual level.
Luhmann (1971, 1984, and 1986) proposed to consider the
processing of meaning as the autopoietic operation of both social and
psychological systems. Social and psychological systems are both structurally
coupled and “interpenetrate” each other reflexively (Luhmann, 1977). Interpenetration
adds an operational dimension to the structural coupling between social and
psychological systems (Luhmann, 1988 [1995a, at p. 51; 2002, at p. 182]). In
this study, we submit operationalizations of these various concepts in terms of
the theory and computation of anticipatory systems (Rosen, 1985; Dubois, 1998a;
Leydesdorff, 2008, 2009).
Meaning can first be provided to events by reflexive systems
(e.g., observers) from the perspective of hindsight (Rosen, 1985). Human
languages allow additionally for the construction and exchange of models using
metaphors (Luhmann, 1995a, at p. 44; 2002, at p. 175; Leydesdorff &
Hellsten, 2005). Metaphors enable us to communicate meaning (Lakoff &
Johnson, 1980; Hesse, 1988; Maasen & Weingart, 1995). New meaning can also be
generated as a result of inter-human communication (Schütz, 1932; Mead, 1934).
When expectations are exchanged and interact, one can expect the development of
a non-linear dynamics of meaning-processing on top of the information exchanges
(MacKay, 1969; Maturana, 2000). Meaning is interactively and recursively
reconstructed, but in an intentional mode, i.e., with reference to a future
state (Husserl, 1929; Schutz, 1975).
The perspective of hindsight—that is, entertaining a model
which provides meaning to the modeled system (Rosen, 1985)—can itself be
modeled as the backward evaluation of a difference equation (Dubois, 1998a). This
has been called “incursion” in order to distinguish it from “recursion” which
follows the arrow of time. For example, the well-known logistic map xt+1 = axt
(1 – xt) can incursively be formulated as
follows:
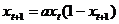 (1)
(1)
and
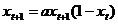 (2)
(2)
In the case of Equation 1, the selection pressure (1 – x)
on the variation (x) develops synchronously with the recursive development
of the system. For example, markets select technological innovations in terms
of current prices, while competing technologies can be expected to develop with
reference to their previous states. Equation 2 has two roots: x = (a – 1)/a,
and x = 0. These roots are the steady states of the logistic
equation and the models of anticipatory systems that can be derived from it.
By considering the future states as the drivers of the
system in the present, three hyper-incursive equations can additionally be
formulated:
 (3)
(3)
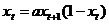 (4)
(4)
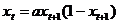 (5)
(5)
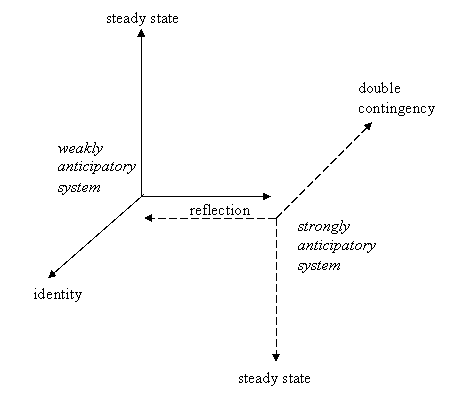
These equations model a “strongly anticipatory” system. While
“weakly anticipatory” systems entertain models of themselves, strongly
anticipatory ones use expectations to construct their current state. In other
words, the incursive and hyper-incursive analogues of the logistic equation
provide us with a model of how social systems of expectations can be reconstructed
on the basis of interactions among weakly anticipatory systems (Leydesdorff
& Dubois, 2004).
The incursive model
Using Equation 1, one can simulate a modeling system
(Leydesdorff, 2005). One can also consider the modeling system as an observer
(Spencer-Brown, 1969). The model appreciates the modeled system by filtering
the noise, as can be understood by rewriting as follows:
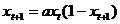 (1)
(1)
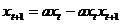 (1a)
(1a)
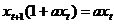 (1b)
(1b)
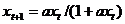 (1c)
(1c)
Unlike the logistic map, this anticipatory system does not
bifurcate, but develops along the curve of the steady state—x = (a
– 1)/a)—for all values of a. Figure 1 shows the results of the
simulation. The biological variation bifurcates and increasingly generates
chaos for 3.57 < a < 4, while the anticipatory system grows continuously
to the limit value of x = 1 with increasing values of a.
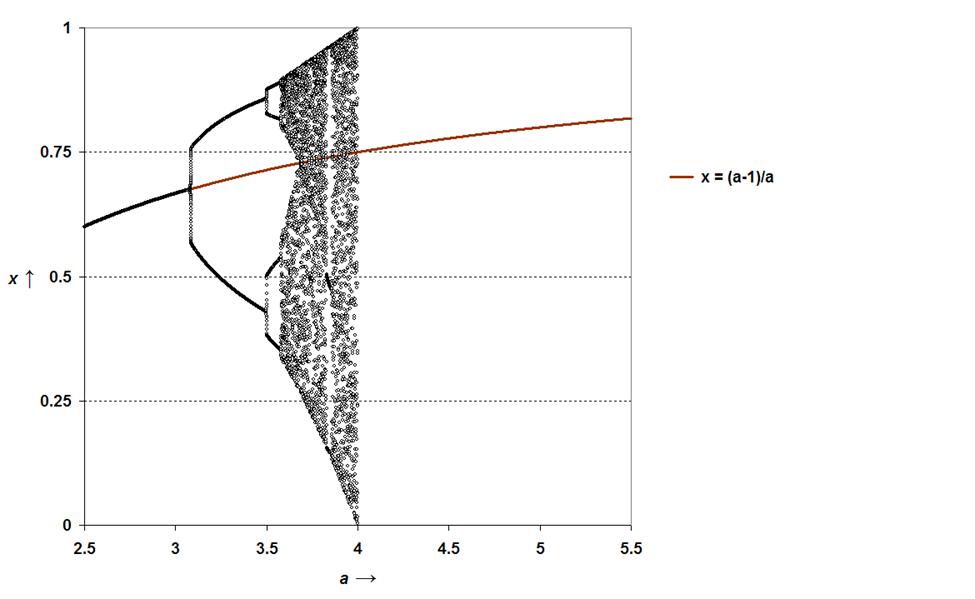
Figure 1: The steady state of the weakly anticipatory system.
The line penciled into Figure 1 can be considered as an emerging
axis stabilizing an identity among the reflections at each moment of time. The
weakly anticipatory system provides meaning to the events by integrating them in
both the biological domain (a < 4; e.g., bodily perceptions) and the social
domain of meaning-processing (a ≥ 4). This integration of the
different representations functions as a linchpin for developing a strongly
anticipatory system in the cultural (i.e., non-natural) domain of meaning-processing
(a ≥ 0.4).
The hyper-incursive model
The hyper-incursive Equations 3, 4, and 5 provide us with the
three building blocks of a non-linear dynamics of expectations (May, 1976; May
& Leonard, 1975). Equation 3 first evolves into x = (a – 1)/a.
This is equal to the steady state of the weakly anticipatory system. In
other words, weak anticipation can be considered as one of the sub-dynamics of a
strongly anticipatory system. In Luhmann’s terminology, one could perhaps consider
this correspondence as the re-entry of the first-order observation into the
system of second-order observations (Luhmann, 1993 [1999]).
Equation 4 evolves into xt+1 = (1/a)
[xt / (1 – xt)]. This routine formalizes
the reflexive operation: when xt > [a / (1 + a)]
a pulse is generated which first overshoots the value of one,
but then generates a negative value (Figure 2). The negative value provides a
mirror image of the representation at a specific moment in time, and thus
allows for a reflection. Reflection enables a system to bounce a communication.
Note that the combination of Equations 3 and 4 provides the weakly anticipatory
system with reflexive access to the meaning processing at the supra-individual
level.
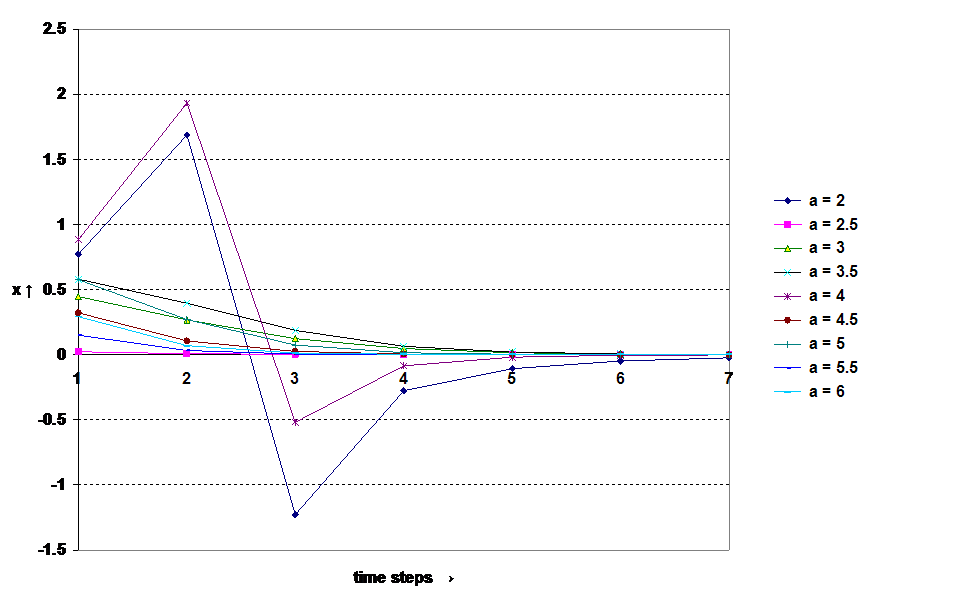
Figure 2: Simulation
of Equation 4.
Equation 5 invokes the perspective on social systems as
introduced here: Ego and Alter are bound by a double
contingency of expecting each other to be first historical and biological, but
secondly reflexive and intentional (Parsons, 1968; Luhmann, 1984). Using the
second layer of the double contingency, Ego in the present (xt)
no longer refers to oneself as an identity which is rooted in the past, but to
oneself in a future state (xt+1), that is, as an Alter Ego.
The non-linear interactions among expectations can generate the social world as
a system different from psychological ones. However, this social system of
expectations remains structurally coupled to psychological ones; otherwise, nobody
would be able to articulate the “horizons of meaning” which are generated.
Social coordination mechanisms are only perceptible by human
beings after a reflexive turn (Giddens, 1984). Let us now show that reflexive
decision-making is endogenous to the social system of expectations (Dubois,
1998b). Equation 5 can be rewritten as follows:
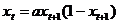 (5)
(5)
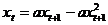


In general, this equation has two solutions:
xt+1 = ½ ± ½ √[1 – (4/a) xt] (6)
Given that 0 ≤ x ≤ 1, the curve x
= 0.5 ± 0.5 √ (1 – (4/a)) for x = 1 sets limits to the
possible values reached by the social system (Figure 3).
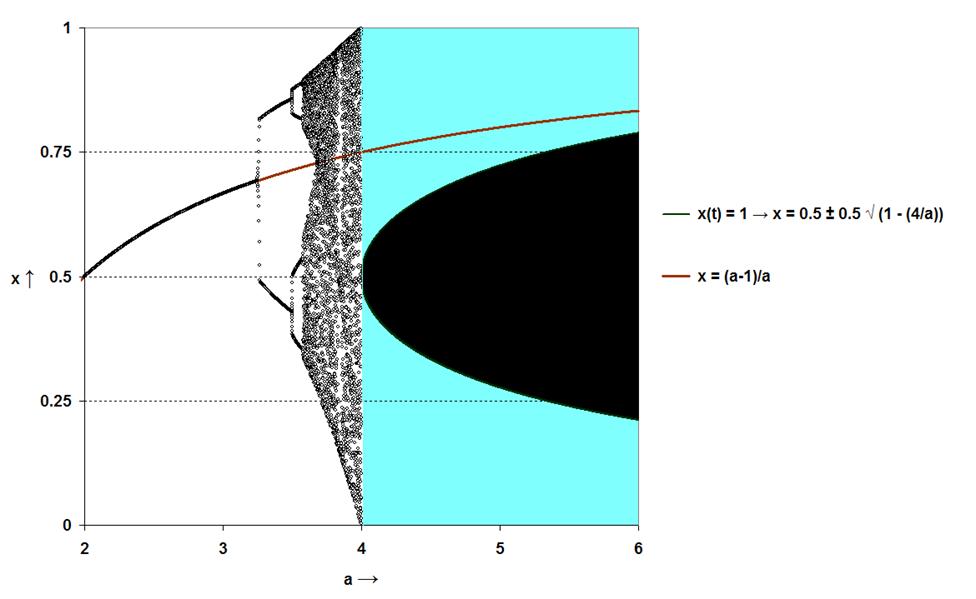
Figure 3: The social system as a result of
hyper-incursion.
For a ≥ 4, two sets of expectations are
generated at each time step depending on the plus or the minus sign in the
equation. After N time steps, 2N future states would
be possible. Thus, the social system of expectations needs continuously a
mechanism for making decisions between options because otherwise this system
would rapidly become overburdened with uncertainty.
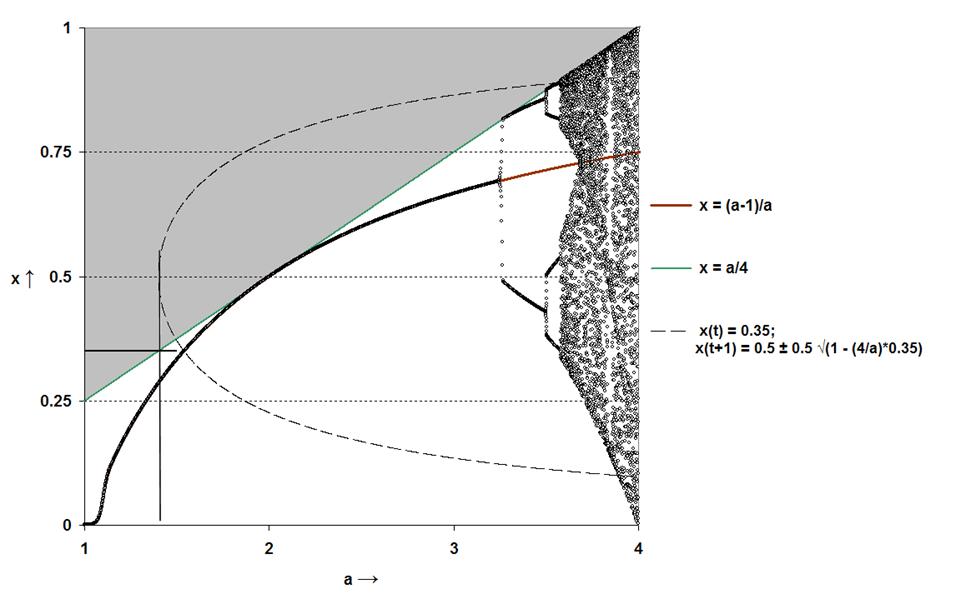
Figure 4: Possible penetrations of the social system
into the biological variation (a < 4).
The term under the root in Equation 6 is positive for xt
≤ a/4: this condition is met for a ≥ 4, but sets a
borderline to the possible penetrations of the social system into the
biological variation (a < 4). In Figure 4, this limitation is elaborated
for a = 1.2 and xt = 0.35. Since in a next step xt+1
= 0.5, and thus larger than a/4 (= 0.3), the strongly anticipatory system
would not be able to proceed with a next step to xt+2.
Expectations cannot operate on expectations for a < 4, but
next states of the natural system can be constructed on the basis of
expectations like in the case of the socio-economic construction of technologies
(Leydesdorff, 2006).
Decisions and historical
trajectories
The social system cannot further be developed at a ≥
4 without a form of agency taking decisions because of the continuous
production of uncertainty by the hyper-incursive mechanism (Eq. 5). Luhmann
(2000) identified decisions as structuring organizations. Although this
reflexive capacity is conceptualized by Luhmann as endogenous to the social
system, organizations can also be attributed with institutional agency. A
psychological system can then perhaps be considered as the minimal unit of
reflection for making choices (Habermas 1981; Leydesdorff 2000, 2001). If
decisions are socially further organized—for example, by using decision
rules—an institutional layer can increasingly be shaped. The institutional
layer provides a retention mechanism for the next round of expectations (Aoki
2001; Luhmann 2000). Thus, the social system is dually layered as a forward-moving
retention mechanism and sets of possible expectations which flow through the
networks. These “horizons of meaning” are not given, but continuously undergoing
reconstruction (Luhmann 1990, 2002).
The strongly anticipatory system is not autonomous, but autopoietic
since structurally coupled to the layer of decision-making by weakly
anticipatory agents (Collier 2006). Because the equations are derived from the
same logistic equation, the steady states of the systems are equal: the systems
are not only structurally coupled, but also coupled in terms of their reflexive
operation. As noted, Luhmann (1977, 1995a [2002]) reserved the term
“interpenetration” (Parsons, 1968, at p. 473; Luhmann, 1978) for this
additional dimension in the coupling. Interpenetration is possible because of
the evolutionary achievement of developing human language ((Luhmann, 1995a, at
p. 44; 2002, at p. 175; Leydesdorff 2002). Communication provides the strongly
anticipatory system with one degree of freedom more than the weakly
anticipatory ones. Unlike individuals, the social system can be expected
to remain distributed. The uncertainty in the double contingency (Equation 5)
is reproduced by the system and this makes the hyper-incursive anticipations unstable
flows of communication.
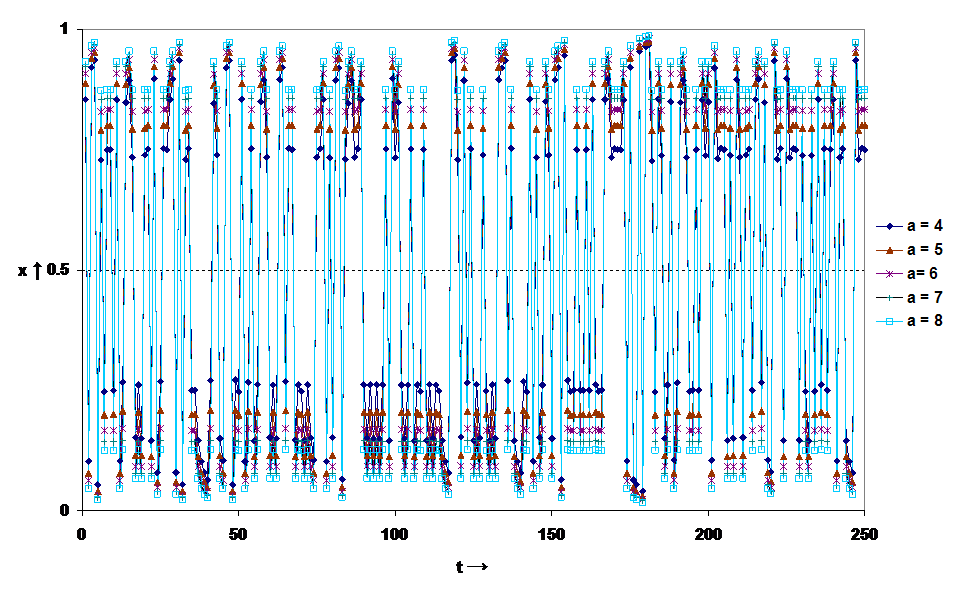
Figure 5: Trajectory of a social system based on
random decisions of the decision-making units, for various values of the
bifurcation parameter a.
Figure 5 shows a simulation of trajectories for different
values of a where decisions are taken randomly. The system
sometimes dwells in a specific state. Next-order mechanisms like
institutionalization can be expected to stabilize these configurations because
decisions are then no longer taken randomly (Dubois 2001).
Figure 6: The relations among the various subdynamics
Figure 6 summarizes the subdynamics which were distinguished
above. While the weakly anticipatory system tends to integrate representations
by organizing them into an identity, the strongly anticipatory one is based on
uncertainty contained in the distribution. However, this additional degree of
freedom cannot be used by this system without the mediation of agency using the
additional coupling which is provided reflexively. The two systems are not only
structurally coupled as systems, but also in terms of the reflexive operation
of providing meaning to each other’s operations.
Conclusions
The incursive and hyper-incursive equations provide us with models
of meaning-processing at the individual and supra-individual levels. The
incursive equation models the operation of a modeling system in relation to the
modeled one. This corresponds with Rosen’s (1985) definition of an anticipatory
system. The hyper-incursive equations enable us to model the structural
coupling between historical phenomena and emerging horizons of meaning. Since
hyper-incursion and incursion can recursively be applied to the results of the
operations, the modeling and simulation of further codification of meanings
(e.g., into discursive knowledge) becomes feasible.
At the theoretical level, these algorithmic results can be
appreciated with reference to the Habermas-Luhmann discussion (Habermas &
Luhmann 1971) about communicative competencies (of agency) versus
communication systems. The opposing perspectives in this debate can be
considered as providing two geometrical metaphors to the algorithmic operations
of the social system (Leydesdorff, 2000). Geometrical metaphors generate their
respective “blind spots.” Habermas (1981), for example, shares with Giddens
(1984) the assumption that the operation of the social system of expectations
remains necessarily “virtual” and therefore unspecifiable. Luhmann (1984)
specified this operation theoretically by proposing a deliberate abstraction
from human agency (Luhmann, 1995b).
Luhmann’s theory could be used fruitfully as a heuristics in
these simulations. However, our results suggest that because of the complex
relation between structural coupling between the two types of systems, and
the interpenetrating reflection of their mutual operations, decision-making and
therefore agency can be considered as back on stage. This is endogenous to the
system and can also be appreciated as a re-entry. In Luhmann’s theory, the
social system is considered as operationally closed and this organizational
reflection can only be formulated as re-entry. However, the alternative
perspective of considering the system as semi-autopoietic (since dependent for
its further development on human or organizational reflections and agency)
remains also possible. An advantage of this latter perspective may be that it
is more compatible with mainstream sociological theorizing by appreciating
reflexive agency while keeping the surplus value of Luhmann’s theory.
References
Aoki M. 2001. Towards
a Comparative Institutional Analysis. Cambridge, MA: MIT Press.
Collier J. 2006.
Conditions for Fully Autonomous Anticipation. In Computing Anticipatory
Systems, CASYS’05, Dubois DM (ed). Melville, NY: American Institute of
Physics Conference Proceedings; 839: 282-289.
Dubois DM. 1998a.
Computing Anticipatory Systems with Incursion and Hyperincursion. In Computing
Anticipatory Systems, CASYS-First International Conference, Dubois DM (ed).
Woodbury, NY: American Institute of Physics Conference Proceedings; 437:
3-29.
Dubois DM. 2001.
Theory of Incursive Synchronization and Application of a Chaotic Epidemic. International
Journal of Computing Anticipatory Systems, 10: 3-18.
Dubois, DM.
1998b. Emergence of Chaos in Evolving Volterra Ecosystems. In Evolutionary
Systems: Biological and Epistemological Perspectives on Selection and
Self-Organization, Van de Vijver G, Salthe S, Delpos M. (eds). Dordrecht, Boston, London: Kluwer Academic Publishers; 197-214.
Giddens A. 1984. The
Constitution of Society. Cambridge: Polity Press.
Habermas J,
Luhmann N. 1971. Theorie der Gesellschaft oder Sozialtechnologie. Frankfurt a.M.: Suhrkamp.
Habermas J. 1981.
Theorie des kommunikativen Handelns.
Frankfurt a.M.: Suhrkamp.
Hesse M. 1988.
The Cognitive Claims of Metaphors. Joural of Speculative Philosophy, 2(1):
1-16.
Husserl E. 1929. Cartesianische
Meditationen und Pariser Vorträge. [Cartesian meditations and the Paris lectures.]. The Hague: Martinus Nijhoff, 1973.
Lakoff G, Johnson
M. 1980. Metaphors we live by: University of Chicago Press Chicago.
Leydesdorff
L, Dubois, DM. 2004. Anticipation in Social Systems: The Incursion and
Communication of Meaning. International Journal of Computing Anticipatory
Systems, 15: 203-216.
Leydesdorff L, Hellsten I. 2005. Metaphors and Diaphors in Science Communication: Mapping the Case of
‘Stem-Cell Research’. Science Communication, 27(1): 64-99.
Leydesdorff L.
2000. Luhmann, Habermas, and the Theory of Communication. Systems Research
and Behavioral Science, 17(3): 273-288.
Leydesdorff L.
2001. A Sociological Theory of Communication: The Self-Organization of the
Knowledge-Based Society. Parkland, FL: Universal Publishers; at http://www.universal-publishers.com/book.php?method=ISBN&book=1581126956.
Leydesdorff L. 2002.
The Communication Turn in the Theory of Social Systems. Systems Research and
Behavioral Science, 19(2): 129-136.
Leydesdorff L.
2005. Anticipatory Systems and the Processing of Meaning: A Simulation Inspired
by Luhmann's Theory of Social Systems. Journal of Artificial Societies and
Social Simulation, 8(2), Paper 7, at http://jasss.soc.surrey.ac.uk/8/2/7.html
[5 April 2007].
Leydesdorff L.
2006. The Knowledge-Based Economy: Modeled, Measured, Simulated. Boca Rota, FL: Universal Publishers.
Leydesdorff L. 2008.
The Communication of Meaning in Anticipatory Systems: A Simulation Study of the
Dynamics of Intentionality in Social Interactions. In Proceedings of the 8th
International Conference on Computing Anticipatory Systems CASYS'07, Dubois
DM (ed). Melville, NY: American Institute of Physics Conference Proceedings (In
print).
Leydesdorff L. 2009.
The Non-linear Dynamics of Meaning-Processing in Social Systems. Social
Science Information, 48(1), forthcoming.
Luhmann N. 1971.
Sinn als Grundbegriff der Soziologie. In Theorie der Gesellschaft oder
Sozialtechnologie, Habermas J, Luhmann N. (eds). Frankfurt a.M.: Suhrkamp;
25-100
Luhmann N. 1977.
Interpenetration – Zum Verhältnis personaler und sozialer Systeme. Zeitschrift
für Soziologie, 6(1): 62-76.
Luhmann N. 1978.
Interpenetration bei Parsons. Zeitschrift für Soziologie, 7:
299-302.
Luhmann N. 1984. Soziale
Systeme. Grundriß einer allgemeinen Theorie. Frankfurt a. M.: Suhrkamp.
Luhmann N. 1986.
The autopoiesis of social systems. In Sociocybernetic Paradoxes, Geyer
F, Van der Zouwen J. (eds). London: Sage: 172-192.
Luhmann N. 1988.
Wie ist Bewusstsein an Kommunikation beteiligt? In Materialität der
Kommunikation, Gumbrecht HU, Pfeiffer KL. (eds). Frankfurt: Suhrkamp; pp.
884-905.
Luhmann N. 1990.
The Cognitive Program of Constructivism and a Reality that Remains Unknown. In Selforganization.
Portrait of a Scientific Revolution, Krohn W, Küppers G, Nowotny H. (eds). Dordrecht: Reidel; 64-85.
Luhmann N. 1993.
Die Paradoxie der Form. In Kalkül der Form, Baecker D. (ed). Frankfurt a. M.: Suhrkamp; 197-212.
Luhmann N. 1995a.
Soziologische Aufklärung 6. Opladen: Westdeutscher Verlag.
Luhmann N. 1995b.
On the Concepts “Subject” and “Action”. Preface to the English edition. In Social
Systems. Stanford, CA: Stanford University Press; xxxvii-lii.
Luhmann N. 1999.
The Paradox of Form. In Problems of Form, Baecker D. (ed). Stanford, CA:
Stanford University Press; 15-26.
Luhmann
N. 2000. Organisation und Entscheidung. Opladen: Westdeutscher Verlag.
Luhmann N. 2002.
How Can the Mind Participate in Communication? In Theories of Distinction:
Redescribing the Descriptions of Modernity, Rasch W. (ed). Stanford, CA: Stanford University Press; 169–184.
Luhmann N. 2002.
The Modern Sciences and Phenomenology. In Theories of Distinction:
Redescribing the descriptions of modernity, W. Rasch (ed). Stanford, CA: Stanford University Press; 33-60.
Maasen S, Weingart
P. 1995. Metaphors--Messengers of Meaning. Science Communication, 17(1):
9-31.
MacKay DM. 1969. Information,
Mechanism and Meaning. Cambridge and London: MIT Press.
Maturana HR.
1978. Biology of language: the epistemology of reality. In Psychology and
Biology of Language and Thought. Essays in Honor of Eric Lenneberg, G. A.
Miller & E. Lenneberg (eds). New York: Academic Press; 27-63.
Maturana HR.
2000. The Nature of the Laws of Nature. Systems Research and Behavioral
Science, 17: 459-468.
May RM, Leonard
WJ. 1975. Nonlinear Aspects of Competition between Three Species. SIAM Journal of Applied Mathematics, 29(2): 243-253.
May RM. 1976.
Simple mathematical models with very complicated dynamics. Nature, 261(June
10): 459-467.
Mead GH. 1934.
The Point of View of Social Behaviourism. In Mind, Self, & Society from
the Standpoint of a Social Behaviourist. Works of G. H. Mead. Morris CH
(ed). Chicago and London: University of Chicago Press; Vol. 1: 1-41.
Parsons T. 1968.
Interaction: I. Social Interaction. In The
International Encyclopedia of the Social Sciences, Sills DL (ed.). New York: McGraw-Hill; Vol. 7: 429-441.
Rosen R. 1985. Anticipatory
Systems: Philosophical, mathematical and methodological foundations. Oxford, etc.: Pergamon Press.
Schütz A. 1932. Der sinnhafte Aufbau der sozialen Welt. Vienna: Springer. [The Phenomenology of the Social World. Walsh G, Lehnert F,
Trans. Evanston, IL: Norwestern University Press, 1967.]
Shannon CE,
Weaver W. 1949. The Mathematical Theory of Communication. Urbana: University of Illinois Press.
Spencer-Brown G.
1969. Laws of Form. London: George Allen and Unwin.
return